Basic Math for Physics

Algebra

Calculations with Significant Figures

Adding & Subtracting:

• Perform the calculation
• Determine the least # of decimal places in problem
• Round answer to that # of decimal places
•         Example:           10.027 g   ® 3 decimal places
•                                  - 1.5     g   ® 1 decimal place
•                                   8.527  g  ® 8.5 g (1 decimal place)
•  

Multiplying & Dividing:

• Perform the calculation
• Determine the least # of sig figures in problem
• Round answer to that # of sig figures
•       Example:         10.027 g   ® 5 sig figs
•                                1.50 mL  ® 3 sig figs
• answer = 6.6847 g/mL ® 6.68 g/mL (3 sig digs)

Scientific Notation

• Scientific Notation—a short hand method of writing numbers using powers of 10.
•  
• Writing scientific notation:
• The decimal point is always moved to after the 1st non-zero number.
• Count the number of times the decimal point is moved and use this as the power of 10.
• “Big” numbers (>1) have positive exponents.  “Small” numbers (<1) have negative exponents.
•       Examples:      1027500.456 ® 1.027500456 ´ 106
•                            0.0007543 ® 7.543 ´ 104
•  
• Reading scientific notation:
• Power of 10 = number of times to move decimal point
• Positive powers = make the number “Big” (>1).  Negative exponents = make the number “Small” (<1)
• Examples:     3.25 ´ 10-6 ® 0.00000325
• 7.2004 ´ 104 ® 7200.4

Math with Exponents

• Calculations with exponents:

Anything to the power of “1” = itself

•                   251 = 25

Anything to the power of “0” = 1

•                   250 = 1

Multiplying (with the same base) à add the powers

•                   32 * 38 = 311

Dividing (with the same base) à subtract the powers

•                   32 ¸ 38 = 3-6

When taking a power of a power à multiply the powers

•                  (32)3 = 36

A negative power puts the number on the opposite side of the fraction & the power becomes positive.

•                   3-2 = 1/32
•  
• Calculations with scientific notation

Adding (with same power of 10):  Add numbers and keep power of 10

•                    2 ´ 103 + 3 ´ 103 = 5 ´ 103

Subtracting (with same power of 10): Subtract numbers and keep power of 10

•                    3 ´ 103 – 3 ´ 103 = -1 ´ 103

Multiplying:  Multiply numbers & add powers of ten

•                    2 ´ 106 · 3 ´ 103 = 6 ´ 1018

Dividing:  Divide numbers & subtract powers of 10

•                    2 ´ 106 ¸ 3 ´ 103 = 0.67 ´ 103

Taking it to a power:  Take the number to the power and multiply the power of 10 by the power

•                   (2 ´ 103)3 = 8 ´ 109

Roots:  Take the number to the root and divide the power of 10 by the root

•                   Ö(3 ´ 102) = Ö3 ´ 101

Trig functions

•      
•     
•    

Quadratic Equation

   where

 

            Example:      If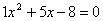 

                               Then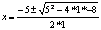  

Calculator Survival

• Always use the ¸ key to designate a number is on the bottom of an expression.
  
• Always use the EE (or EXP) key to enter scientific notation.
  
• Always use parenthesis around addition or subtraction when combining it with other operations
• To make something negative (when taking the number to a power), keep the negative outside of the parenthesis.